About this Product
Tiling the plane with shapes is a problem that dates at least to the Greeks.
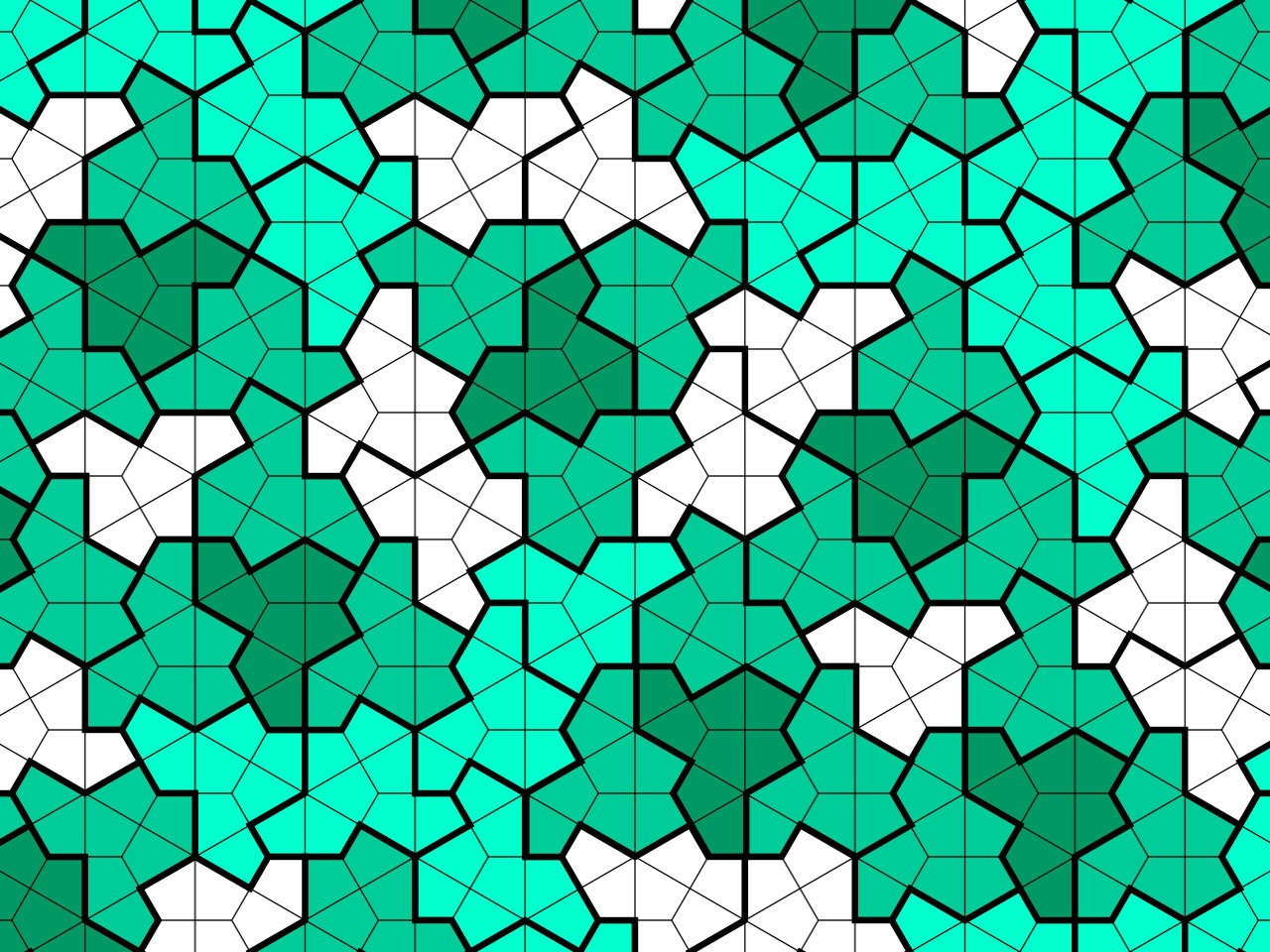
The problem is simple: can you cover an infinite flat surface, a geometric plane, with a particular set of fixed-shaped tiles? The specific subproblem that Smith, et. al. published a solution for in March 2023 is more subtle: with a single tile shape, can you cover a plane with an aperiodic pattern?
The key is "aperiodic". A square tile can cover the plane in a repeated pattern -- a simple grid -- but, this tiling is periodic. If a duplicate of the tiling is laid on top of the original and moved over by one tile, it lines up perfectly with the original. What Smith et. al. discovered, and proved, is that a single tile shape can cover the plane so that the pattern never repeats. Ever.
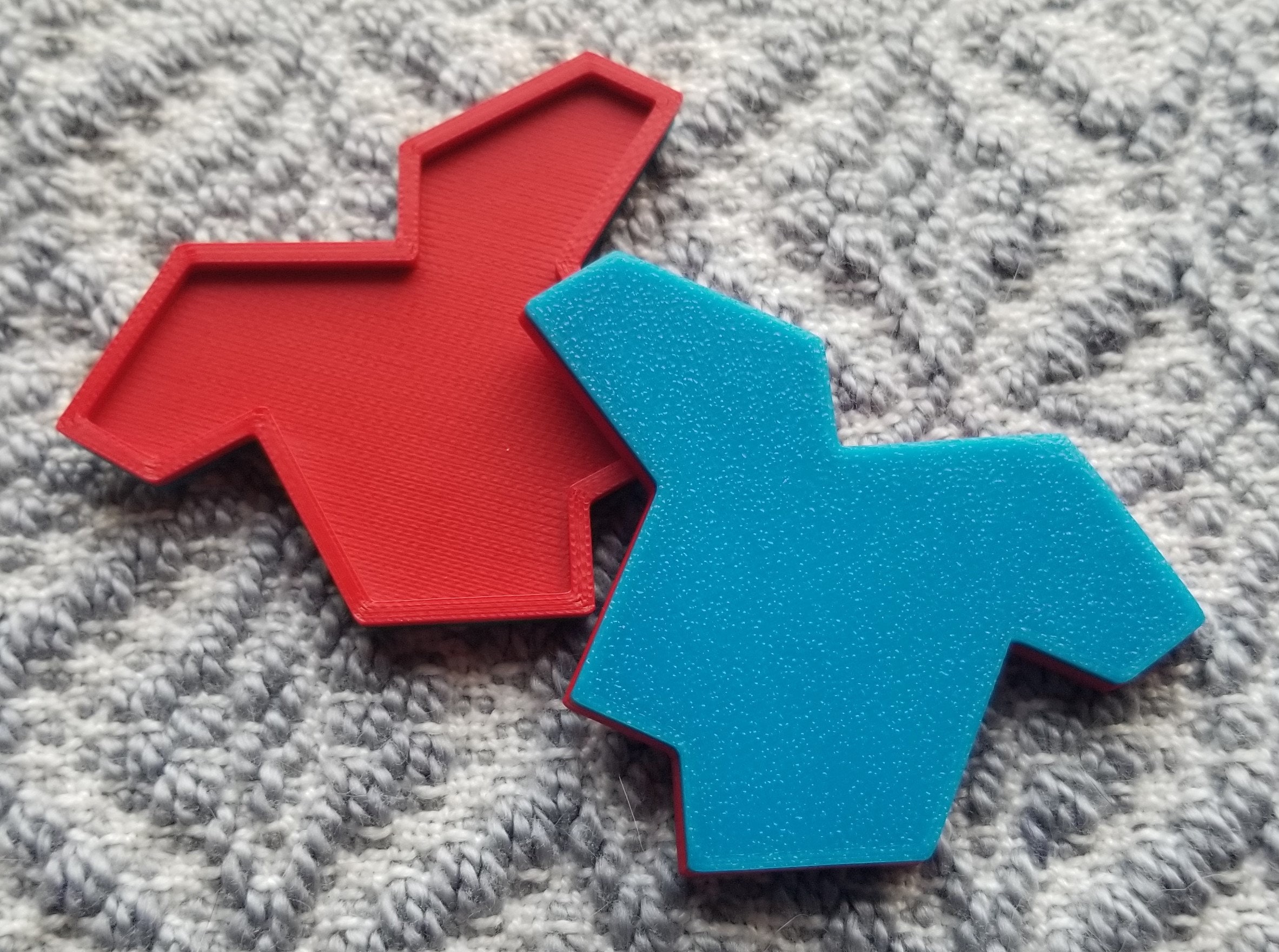
To help explore this, I 3D-printed tiles with the shape they discovered. I have been exploring them with friends, and am offering them here for you to explore.
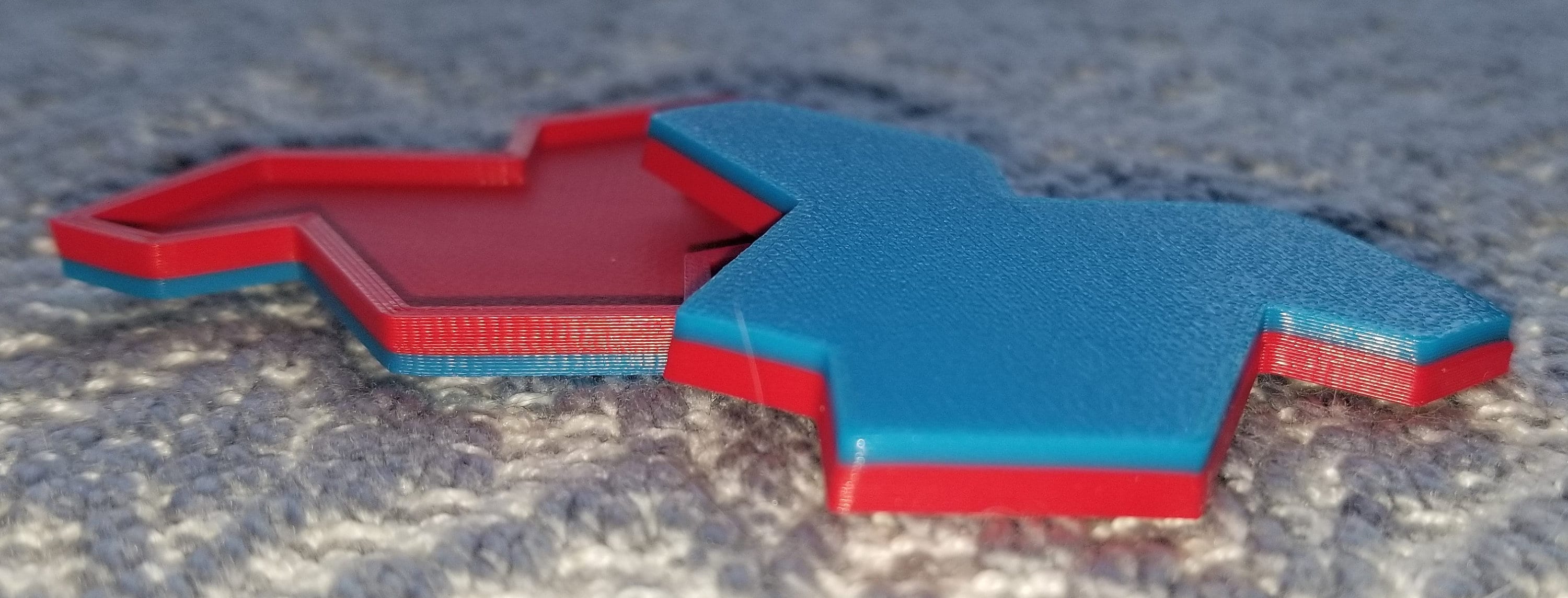
The tiles are printed of safe, corn-based PLA plastic. They are red on one side and blue on the other side because to tile the plane, some tiles in the pattern need to be flipped. The tiles are just over 2 inches between the extreme points and about 1/8" thick. To make them easier to place and move around, each tile is slightly smaller (0.005" from each edge) than the theoretical size.
I package them in sets of 25 -- enough to experiment with the process. To fully tile the plane would require an infinity of tiles, which I can not supply.
If you need different colors or sizes send me a note.
For more information, check out the Wikipedia article on non-periodic tiling: https://en.wikipedia.org/wiki/... , which is also the source of the image.
The original paper is on arXiv:
Smith, David; Myers, Joseph Samuel; Kaplan, Craig S.; Goodman-Strauss, Chaim (2023-03-19). "An aperiodic monotile". arXiv:2303.10798 (https://arxiv.org/abs/2303.10798)
For other aperiodic tilings, I have several other tile options, including the Spectre tiles, Penrose tiles, and magnetic variations. Please check my other listings for the details.
The photos show tiles is blue and pink. If you want these, please send me a note so I can make the right tiles for you.
Carvings by Carl
Meet the Maker
Hello. I am Carl Mikkelsen. I make custom Challah boards, cutting boards, and engagement ring presentation boxes. I also make math exploration tools for planar tilings.
Enjoy my listings, and please message me about your custom projects. I thrive on making new things based on your vision and my abilities.
How it’s Made
These are 3D printed in two colors of PLA. Einstein tiles require that some be flipped. Flipped tiles are the other color.
Shop Policies
Custom work is not returnable. If the work is defective, I will remake or correct it, and may require that ship it back to me for repair.
Work from stock is returnable within 30 days. Contact me and I will give you return instructions.
My goal is to always leave you content with the outcome.