About this Product
Tiling the plane is a problem faced every time we cover a floor or walls with tiles.
Most tiles are symmetrical, and easily cover a flat surface, in mathematical term, a plane.
Greek mathematicians discovered all of the basic shapes that can be used to completely cover a plane. These include rectangles, triangles, rhombuses, and hexagons. They proved they had found them all, but they had only considered periodic tilings.
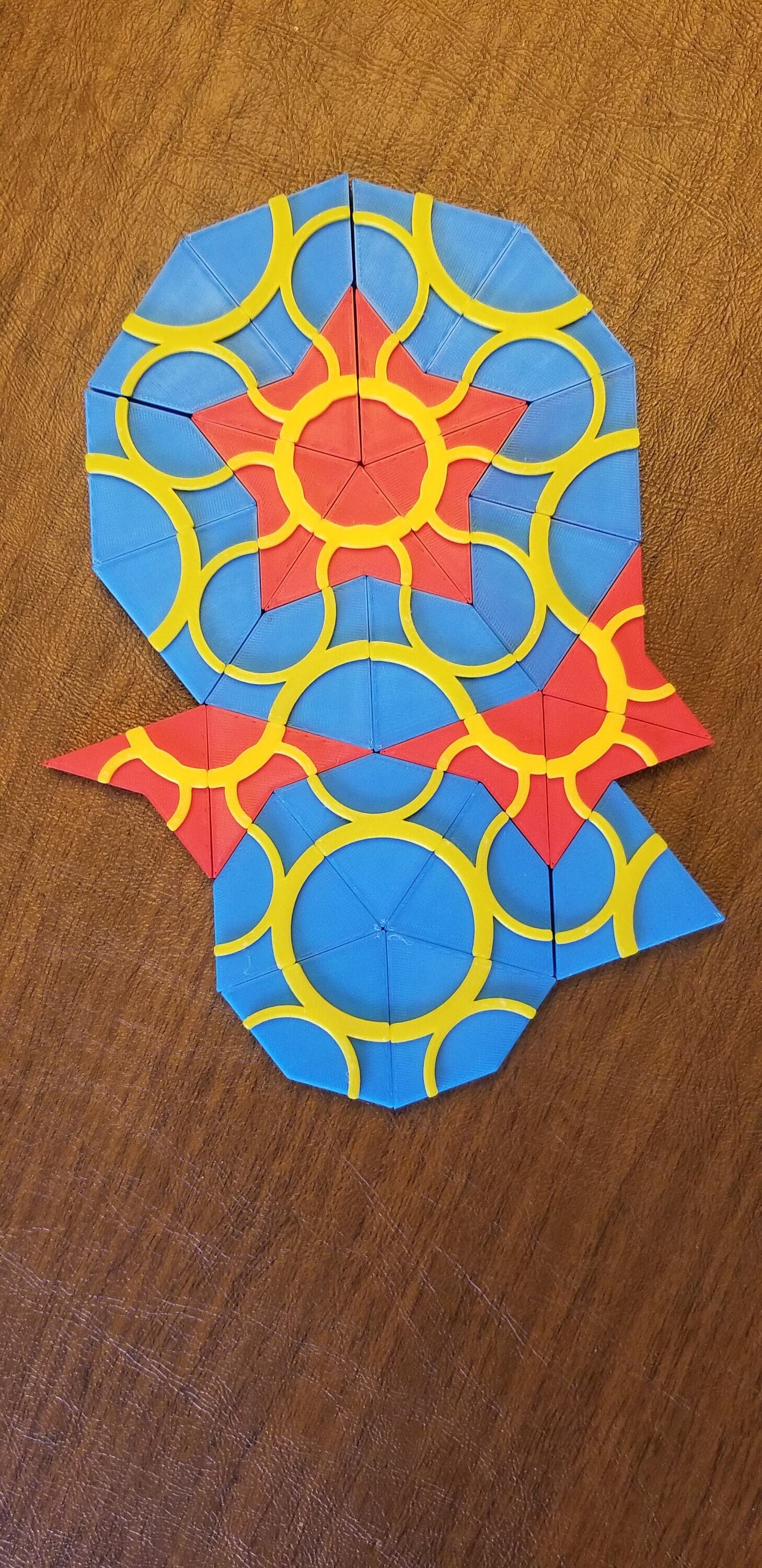
But, aperiodic tiles are a completely new game. Dr. Roger Penrose, mathematician, physicist, and deep thinker, found tiles that would cover the plane but with no true symmetries, but it did show an unexpected five-fold near symmetry.
I could try to explain more, but Wikipedia has it more complete, and better: https://en.wikipedia.org/wiki/Penrose_tiling
These tiles were based on the dart and kite design with overlaid arcs.
I package 16 kites and 10 darts in each pack of 26 tiles. Overall, the ratio of kites to darts required to cover an area converges on the Golden Ratio, so each package is pretty close to the ideal mixture.
These tiles are thick enough to handle easily. The yellow arcs line up to show that the placement constraints are satisfied, and create their own pattern as the tiled area grows.
Carvings by Carl
Meet the Maker
Hello. I am Carl Mikkelsen. I make custom Challah boards, cutting boards, and engagement ring presentation boxes. I also make math exploration tools for planar tilings.
Enjoy my listings, and please message me about your custom projects. I thrive on making new things based on your vision and my abilities.
How it’s Made
I 3D print these tiles using a multi-material printer with three colors of PLA plastic. The Kites are blue. The Darts are red. The thick and thin arcs which constrain placements are yellow.
Shop Policies
Custom work is not returnable. If the work is defective, I will remake or correct it, and may require that ship it back to me for repair.
Work from stock is returnable within 30 days. Contact me and I will give you return instructions.
My goal is to always leave you content with the outcome.